Rafi Ahammed

The blog author is a dedicated PhD scholar at NIT Jalandhar and has completed a Master's from NIFT New Delhi. With over a decade of experience in the textile industry, he specializes in various sectors, including home furnishings, velvet, carpet, bed sheets, suiting, and shirting. He has excelled in roles such as shift in charge, manufacturing manager, and training manager. Notably, he has successfully empowered Omani women in textiles through a government-sponsored training program. Passionate about the industry, they are committed to advancing sectoral excellence through analytical thinking, innovation, and continuous improvement. He possesses a strong desire for learning, tackling challenges, and collaborating effectively in diverse teams, making him a valuable asset to organizations with shared vision and values.

Soft Tissue Replacement Implants

by

Rudra Narayan SahaResearch ScholarDr B R Ambedkar National Institute of Technology, Jalandhar, Punjab, IndiaEmail Id – rudenarayansaha37469@gmail.com 1. Introduction Synthetic polymers are the main reason why soft tissue implants have been so successful. This is mostly because polymers can be made to match the physical and chemical properties of soft tissues. In addition, polymers can … Read more

Identifying Textile Fibers Using a Burning Test

by

The burning test is a traditional method of identifying different types of textile fibers based on their behavior when exposed to a flame. Each fiber type exhibits distinct characteristics during combustion, such as the color of the flame, odor, residue, and whether it melts or continues to burn after the flame is removed. In this … Read more

Denim Finishing using Laser Technology- A detailed study on the working process of laser machines

by

Sourav Ghosh Operation AnalystClassic Fashion Apparel Ltd, JordanEmail: souravghosh090@gmail.com Introduction:  Industry is moving toward 4.0, by matching the foot step garment industry is also moving toward 4.0. Denim washing is also not behind in this race. Denim washing is moving toward sustainability. Laser is a milestone to that path only. Making a pair of denim … Read more

Polyamide: Preparation, Properties, Types, Advantages, Disadvantages, and Applications

by

Introduction Polyamides are polymers formed by the reaction between carboxylic acids and amines, producing a chain linked by amide (-CO-NH-) bonds. These polymers can be naturally occurring, such as proteins, or synthetically produced, like nylon. Polyamides were first commercialized by the American company DuPont in 1938. Natural polyamides include wool and silk, while synthetic types … Read more

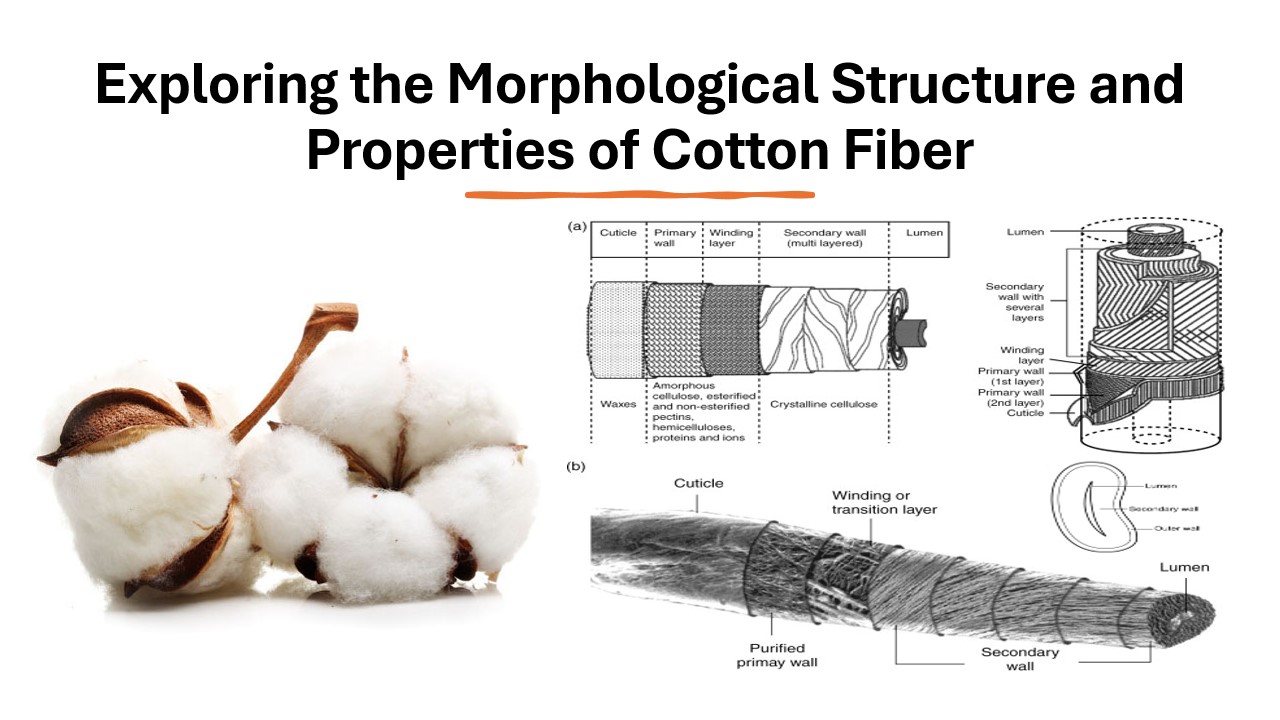
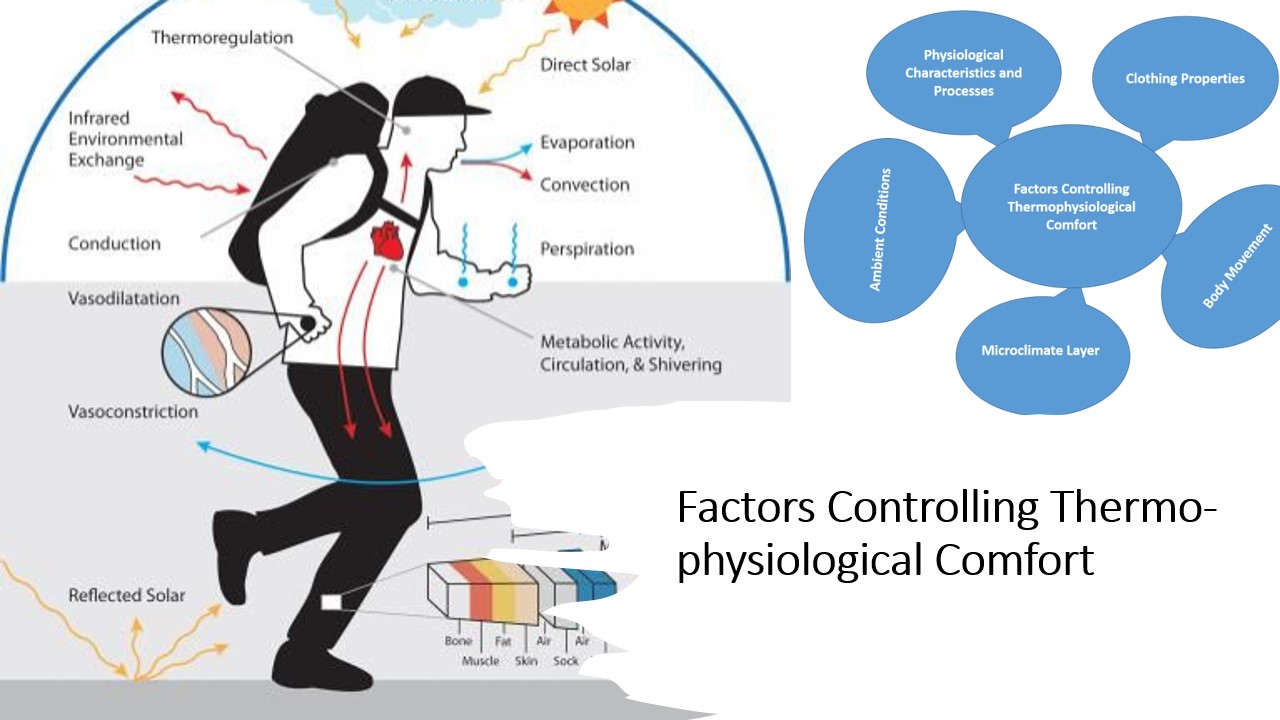
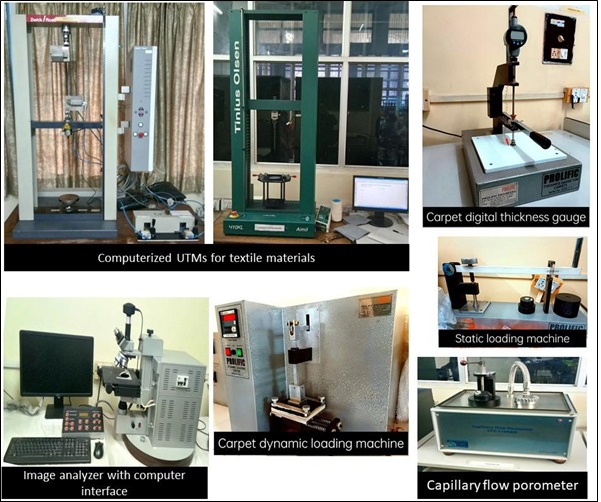
The Diverse Applications of Nonwoven Fabrics: Revolutionizing Industries

by

Nonwoven fabrics have emerged as a game-changer across various industries, owing to their versatility, cost-effectiveness, and unique properties. Unlike traditional textiles, nonwoven fabrics are not woven or knitted; they are produced by bonding or entangling fibres using mechanical, chemical, or thermal techniques. This innovative production process opens the door for applications that traditional textiles cannot … Read more

Exploring the Morphological Structure and Properties of Cotton Fiber 

by

Introduction:  Cotton, one of the most widely used natural fibers, has a unique structure that imparts a variety of beneficial properties. Understanding these structural characteristics is essential for appreciating why cotton is so valued in the textile industry. Cotton fiber, renowned for its natural comfort and versatility, owes much of its performance to its unique … Read more

12 Segments of Technical Textiles: Innovations & Applications

by

Introduction Technical textiles are defined as textile products and materials manufactured primarily for their technical and performance properties rather than for aesthetic or decorative purposes. These specialized textiles have become indispensable in various industries, offering solutions that enhance functionality, safety, and efficiency. With applications spanning from agriculture to construction, healthcare, and transportation, technical textiles are … Read more

A Comprehensive Guide to Different Types of Textile Weaves

by

Textiles are an integral part of our lives, from the clothes we wear to the furnishings that adorn our homes. A fundamental aspect of textiles is the weave, which refers to the way yarns are interlaced to create fabric. Each weave type has its unique characteristics, uses, and aesthetic appeal. This blog will explore various … Read more

Factors Controlling Thermo-physiological Comfort

by

Thermophysiological comfort plays a pivotal role in maintaining body temperature balance and overall well-being, particularly in various environmental conditions. This article explores the key factors affecting thermophysiological comfort, emphasizing the interplay between physiological factors, microclimate layers, clothing properties, ambient conditions, and body movement. 1. Physiological Characteristics and Processes Physiological traits such as age, sex, and … Read more